Problem
When there are two straight lines that intersect on the same plane, two straight lines that are parallel to each of them will always intersect.
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
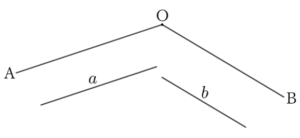
Let $OA$ and $OB$ be two straight lines that intersect at $O$, and let $a∥OA$ and $b∥OB$.
If $a$ and $b$ do not intersect, that is, $a∥b$, then a straight line parallel to one of the parallel lines is also parallel to the other. Thus,
$$a∥OB.$$
Then, there are two straight lines, $OA$ and $OB$, that pass through the point $O$ and are parallel to the straight line $a$.
However, this is irrational because it violates the axiom of parallel lines.
Therefore, $a$ and $b$ are not parallel, but always intersect.
$ $
$ $
$ $
Reference
Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, pp.7-8