Problem
When the two angles of a triangle are unequal, the side opposite the larger angle is longer than the side opposite the smaller angle.
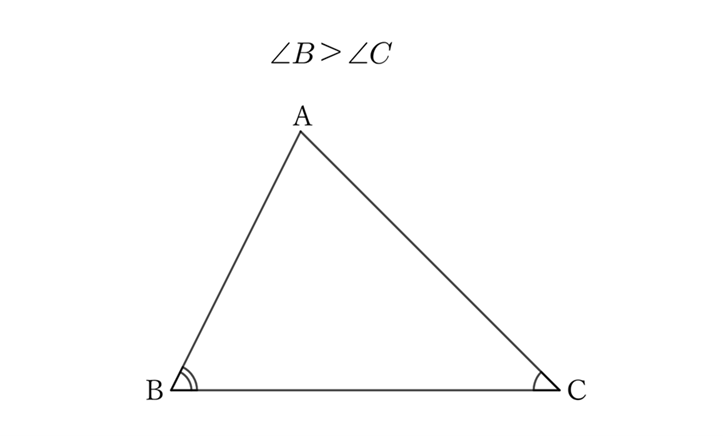
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
In $△ABC$,
$$∠B>∠C.$$
If $AB=AC$, then
$$∠B=∠C,$$
This violates the assumption. Thus,
$$AB≠AC.$$
If $AB>AC$, then according to the previous question $0038$,
$$∠B<∠C,$$
This is also against the assumption. Therefore,
$$AB≯AC,$$
$$∴ \ AB≱AC,$$
$$∴ \ AB<AC.$$
$ $
$ $
$ $
Reference
Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.12