Problem
If we take any point $O$ within $△ABC$ and draw line segments $OB$ and $OC$, we get
$$AB+AC>OB+OC, \qquad and \qquad ∠BOC>∠A.$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
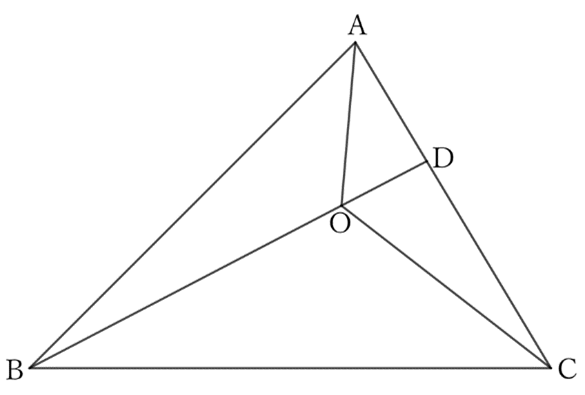
If $BO$ is extended and the point where it intersects with $AC$ is $D$, then in $△ABD$,
$$AB+AD>BD,$$
$$∴ \ AB+AD>OB+OD. \qquad [1]$$
Also, in $△ODC$,
$$OD+DC>OC. \qquad [2]$$
From $[1]$ and $[2]$,
$$AB+AD+OD+DC>OB+OD+OC,$$
$$AB+(AD+DC)>OB+OC,$$
$$∴ \ AB+AC>OB+OC.$$
Also, since the exterior angle of a triangle is greater than any of its inner opposite angles, in
$△ABD$,
$$∠BDC>∠A. \qquad [3]$$
Also, in $△ODC$,
$$∠BOC>∠ODC .\qquad [4]$$
From $[3]$ and $[4]$,
$$∠BDC+∠BOC>∠A+∠ODC,$$
$$∴ \ ∠BOC>∠A. \qquad (∵ ∠BDC=∠ODC)$$
$ $
Reference
Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.13