Problem
In a triangle $ABC$, if $AB>AC$, and we take any point $P$ on the perpendicular $AD$ drawn from the vertex $A$ to the opposite side $BC$, then we have
$$PB-PC>AB-AC.$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
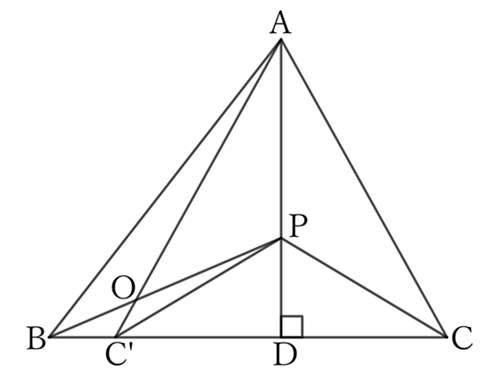
Since $AB>AC$,
$$BD>CD.$$
Therefore, if we take a point $C’$ on $BD$ such that $CD=C’D$, $AC=AC’$ and $PC=PC’$.
If the intersection of $PB$ and $AC’$ is $O$, then
$$OA+OB>AB \qquad and \qquad OC’+OP>PC,$$
$$∴ \ OA+OB+OC’+OP>AB+PC,$$
$$∴ \ (OA+OC’)+(OB+OP)>AB+PC,$$
$$∴ \ AC’+PB>AB+PC,$$
$$∴ \ AC+PB>AB+PC,$$
$$∴ \ PB-PC>AB-AC.$$
$ $
$ $
$ $
Reference
Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.17