Problem
In a triangle $ABC$ such that $∠B=3∠C$, if $BD$ is the perpendicular drawn from $B$ to the bisector of $∠A$, then
$$BD=\frac{1}{2} (AC-AB).$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
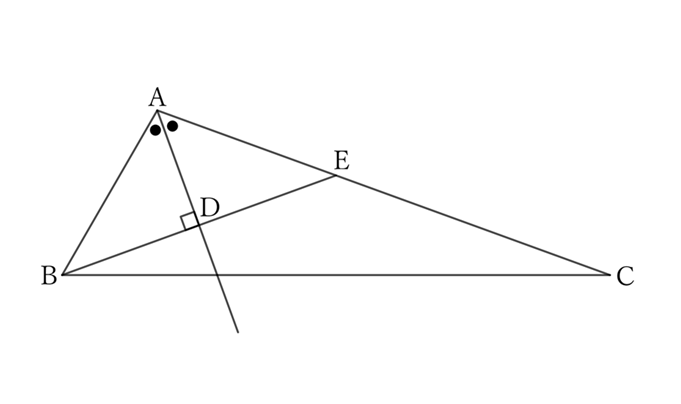
Let $E$ be the point where the extension of $BD$ intersects $AC$.
If $∠C=α$ and $∠BAD=∠EAD=β$, then
$$∠B=3α.$$
Thus,
$$∠A+∠B+∠C=2∠R,$$
$$∴ \ 2β+3α+α=2∠R,$$
$$∴ \ 2α+β=∠R. \qquad [*]$$
Then, $△ABD$ and $△AED$ share the side $AD$, and
$$∠BAD=∠EAD \qquad and \qquad ∠ADB=∠ADE \ (=∠R),$$
$$∴ \ △ABD≡△AED,$$
$$∴ \ AB=AE,$$
$$∴ \ AC-AB=AC-AE=EC.$$
From $[*]$, since $∠ABD=2α$ in $△ABD$,
$$∠EBC=α.$$
Therefore, since $△EBC$ is an isosceles triangle with $E$ as the vertex,
$$EB=EC,$$
$$∴ \ EB=AC-AB.$$
However, since $BD=ED$,
$$BD=\frac{1}{2} (AC-AB).$$
$ $
$ $
$ $
Reference Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, pp.20-21