Problem
Let $AD, \ BE$, and $CF$ be the three medians of $△ABC$, and $G$ be their intersection point. Then,
$$AD+BE+CF<AB+BC+CA<2 \ (AG+BG+CG).$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
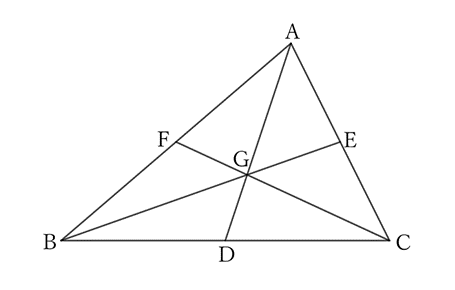
First, from the problem $0098$,
$$AD+BE+CF<AB+BC+CA. \qquad [1]$$
Next, from the diagram,
$$AB<AG+BG, \qquad BC<BG+CG, \qquad and \qquad CA<CG+AG,$$
$$∴ \ AB+BC+CA<2 \ (AG+BG+CG). \qquad [2]$$
From $[1]$ and $[2]$,
$$AD+BE+CF<AB+BC+CA<2 \ (AG+BG+CG).$$
$ $
$ $
$ $
Reference Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.24.