Problem
Let $D$ be the midpoint of the side $AB$ of $△ABC$, and the point $E$ be on the side $AC$ so that $AE∶EC=2∶1$.
Moreover, let $O$ be the intersection of $CD$ and $BE$. Then,
$$BE∶OE=4∶1.$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
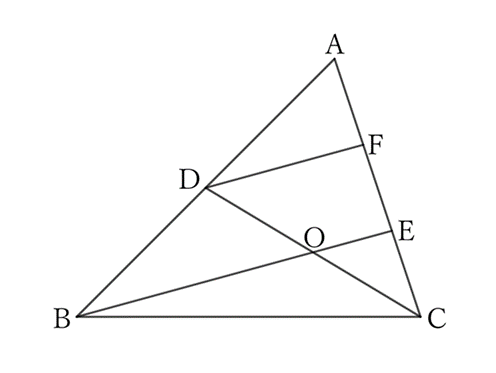
If the midpoint of $AE$ is $F$, then
$$AD=DB \qquad and \qquad AF=FE,$$
$$∴ \ DF∥BC.$$
$$△ABE \sim △ADF \qquad and \qquad AB∶AD=2∶1,$$
$$∴ \ BE∶DF=2∶1. \qquad [1]$$
$$△DFC \sim △OEC \qquad and \qquad FC∶EC=2∶1,$$
$$∴ \ DF∶OE=2∶1. \qquad [2]$$
From $[1]$ and $[2]$,
$$BE:DF:OE=2:1:\frac{1}{2},$$
$$∴ \ BE∶OE=4∶1.$$
$ $
$ $
$ $
Reference Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.26.