Problem
Let $M$ be the midpoint of the base $BC$ of an isosceles triangle $ABC$.
When a line passing through $M$ intersects with the side $AB$ at the point $D$ and with the extension of the side $AC$ at the point $E$,
$$AB+AC<AD+AE.$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
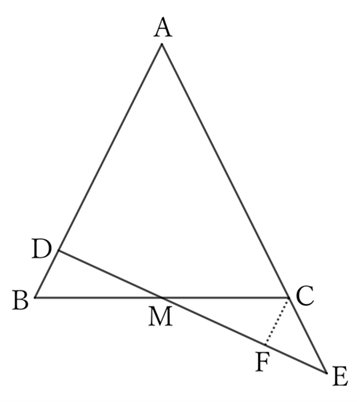
If $F$ is the point where a line parallel to $AB$ passing through $C$ intersects with $DE$, then
$$BM=CM, \qquad ∠BMD=∠CMF\qquad and \qquad ∠MBD=∠MCF,$$
$$∴ \ △BDM≡△CFM,$$
$$∴ \ BD=CF. \qquad [*]$$
$△ADE$ and $△CFE$ share $∠E$, and because $AB∥CF$,
$$∠ADE=∠CFE,$$
$$∴ \ △ADE∼△CFE,$$
$$∴ \ AD∶AE=CF∶CE.$$
However, since $AB=AC$,
$$AD<AE,$$
$$∴ \ CF<CE,$$
$$∴ \ BD<CE, \qquad (∵ \ [*])$$
$$∴ \ AB+AC<(AB-BD)+(AC+CE).$$
$$∴ \ AB+AC<AD+AE.$$
$ $
$ $
$ $
Reference Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.33.