Problem
In a quadrilateral $ABCD$, if the angles of intersection of the bisectors of $∠A$ and $∠B$, and $∠A$ and $∠C$ are $α$ and $β$, respectively, then
$$α=\frac{1}{2} (∠C+∠D) \qquad and \qquad β=\frac{1}{2} |∠B-∠D|.$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
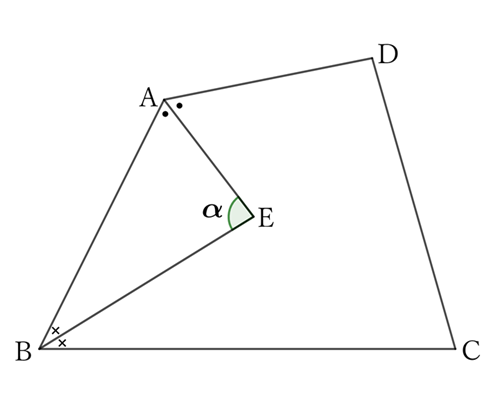
From the diagram,
$$∠A+∠B+∠C+∠D=4∠R. \qquad [1]$$
Furthermore,
$$\frac{1}{2}∠A+\frac{1}{2}∠B+α=2∠R,$$
$$∴ \ ∠A+∠B+2α= 4∠R. \qquad [2]$$
From $[1]$ and $[2]$,
$$2α=∠C+∠D,$$
$$∴ \ α=\frac{1}{2} (∠C+∠D).$$
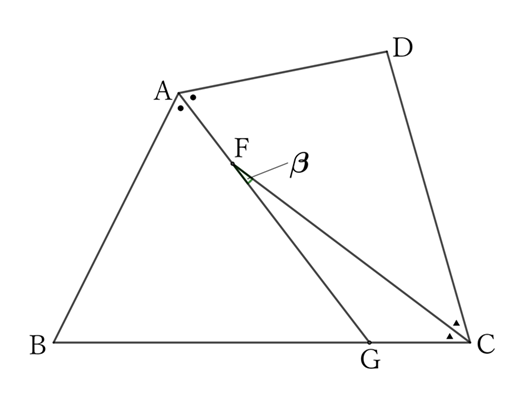
If the intersection point of the bisector of $∠A$ and $BC$ is $G$,
$$\frac{1}{2}∠A+∠B+∠BGA=2∠R,$$
$$∠A+2∠B+2∠BGA=4∠R. \qquad [3]$$
From $[3]-[1]$,
$$∠B-∠C-∠D+2∠BGA=0. \qquad [4]$$
If the intersection point of the bisectors of $∠A$ and $∠C$ is $F$,
the following can be said:
$$∠FCG+∠GFC=∠BGA,$$
$$∴ \ ∠BGA=\frac{1}{2}∠C+β. \qquad [5]$$
Substituting $[5]$ into $[4]$, we get
$$∠B-∠C-∠D+2 \left( \dfrac{1}{2}∠C+β \right)=0,$$
$$2β=∠D-∠B=|∠B-∠D|,$$
$$∴ \ β=\frac{1}{2} |∠B-∠D|.$$
$ $
$ $
$ $
Reference Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.44.