Problem
If the bisectors of tangent angles $∠AOB$ and $∠BOC$ are $OM$ and $ON$ respectively, then
$$∠MON=\frac{1}{2}(∠AOB+∠BOC).$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
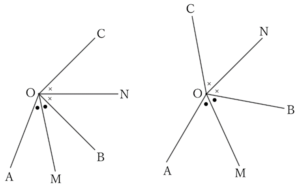
Since $OM$ is the bisector of $∠AOB$, as shown in the figure,
$$∠MOB=\frac{1}{2}∠AOB.$$
Similarly, since $ON$ is the bisector of $∠BOC$,
$$∠BON=\frac{1}{2}∠BOC.$$
Since $∠MON=∠MOB+∠BON$,
$$∠MON=\frac{1}{2}∠AOB+\frac{1}{2}∠BOC,$$
$$∴ \ ∠MON=\frac{1}{2} (∠AOB+∠BOC).$$
$ $
$ $
$ $
Reference
Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.6