Problem
In a triangle $ABC$, let $O$ be the intersection of the bisectors of $∠B$ and $∠C$, and let $M$ and $N$ be the intersections of the straight line passing through $O$ and parallel to $BC$, and $AB$ and $AC$, respectively. Then,
$$MN=|MB-NC|.$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
(1) When $∠B<∠C$
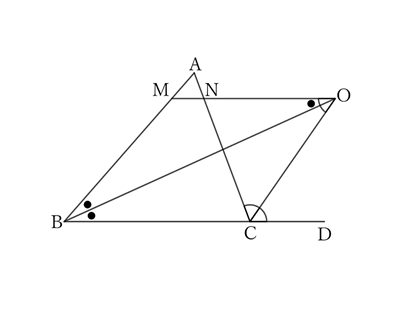
Since $MO∥BC$,
$$∠OBC=∠MOB.$$
By definition,
$$∠MBO=∠OBC,$$
$$∴ \ ∠MOB=MBO.$$
Therefore, since $△MBO$ is an isosceles triangle with $M$ as the vertex,
$$MO=MB.$$
Similarly,
$$∠OCD=∠NOC.$$
By definition,
$$∠NCO=∠∠OCD,$$
$$∴ \ ∠NOC=∠NCO.$$
Thus, since $△NCO$ is an isosceles triangle with $N$ as the vertex,
$$NO=NC.$$
However,
$$MN=MO-NO,$$
$$∴ \ MN=MB-NC. \qquad [1]$$
(2) When $∠B>∠C$
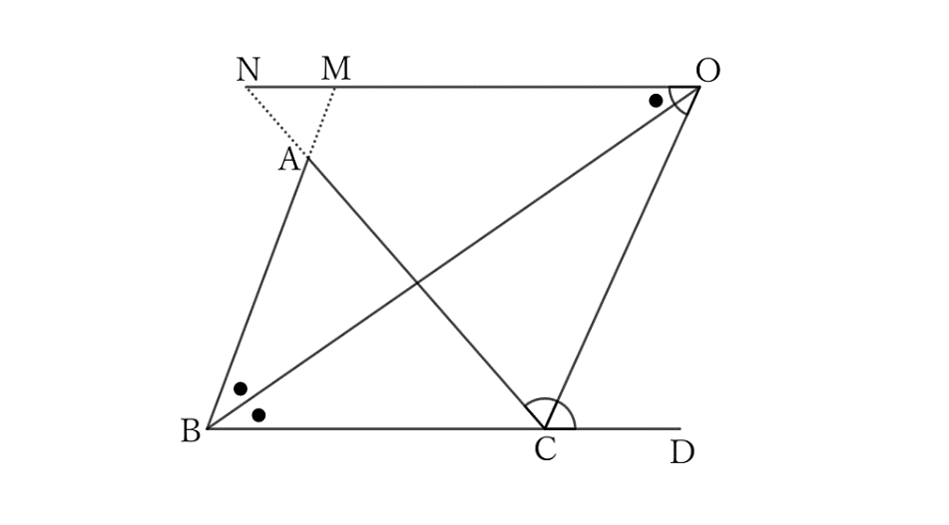
Since $NO∥BC$,
$$∠OBC=∠MOB.$$
By definition,
$$∠MBO=∠OBC,$$
$$∴ \ ∠MOB=∠MBO.$$
Therefore, since $△MBO$ is an isosceles triangle with $M$ as the vertex,
$$MO=MB.$$
Similarly,
$$∠OCD=∠NOC.$$
By definition,
$$∠NCO=∠OCD,$$
$$∴ \ ∠NOC=∠NCO.$$
Thus, since $△NCO$ is an isosceles triangle with $N$ as the vertex,
$$NO=NC.$$
However,
$$MN=NO-MO,$$
$$∴ \ MN=NC-MB. \qquad [2]$$
(3) When $∠B=∠C$

A straight line passing through $O$ and parallel to $BC$ passes through $A$. Therefore,
$$A=M=N,$$
$$∴ \ MN=0.$$
However, since $△ABC$ is an isosceles triangle with $A$ as the vertex,
$$AB-AC=0,$$
$$∴ \ MB-NC=0,$$
$$∴ \ MN=MB-NC=0. \qquad [3]$$
Therefore, in any case of $[1]$ to $[3]$,
$$MN=|MB-NC|.$$
$ $
$ $
$ $
Reference
Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.19