Problem
Let $O$ be the intersection of the bisectors of $∠B$ and $∠C$ of $△ABC$, and let $D$ and $E$ be the feet of the perpendicular lines drawn from $A$ to the straight lines $BO$ and $CO$. Then
$$DE∥BC.$$
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
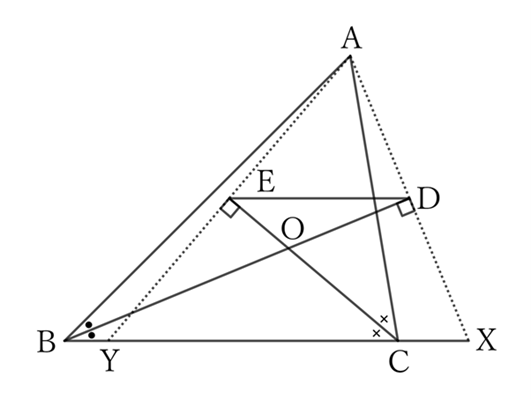
Let the intersection of the extensions of $AD$ and $BC$ be $X$, and the intersection of the extensions of $AE$ and $BC$ be $Y$.
$△BAD$ and $△BXD$ share the side $BD$,
$$∠ABD=∠XBD \qquad and \qquad ∠BDA=∠BDX= \ (∠R),$$
$$∴ \ △BAD≡△BXD,$$
$$∴ \ AD=DX.$$
$△CAE$ and $△CYE$ share the side $CE$,
$$∠ACE=∠YCE \qquad and \qquad ∠CEA=∠CEY \ (=∠R),$$
$$∴ \ △CAE≡△CYE,$$
$$∴ \ AE=EY.$$
Therefore, $△AYX$ and $△AED$ share $∠YAX \ (=∠EAD),$
$$AD:AX=1:2 \qquad and \qquad AE:AY=1:2,$$
$$∴ \ △AYX \sim △AED.$$
Then,
$$∠AED=∠AYX,$$
$$∴ \ ED∥YX,$$
$$∴ \ ED∥BC.$$
$ $
$ $
$ $
Reference Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.22