Problem
If we take a point $C$ on the extension of a line segment $AB$ (or $BA$) and construct equilateral triangles $ACP$ and $BCQ$ on $AC$ and $BC$, the absolute value of the difference of their heights is constant.
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
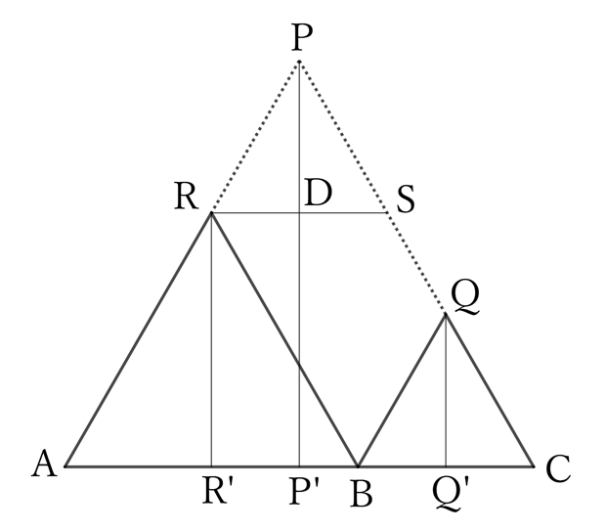
If you place $R$ on $AP$ so that $△ABR$ is an equilateral triangle, and $S$ on $CP$ so that $AC∥RS$, then $RBCS$ is a parallelogram.
Thus,
$$BC=RS.$$
Similarly, $PRBQ$ is also a parallelogram.
Therefore,
$$QB=PR.$$
Since $BC∥RS$ and $QB∥PR$,
$$∠QBC=∠PRS,$$
$$∴ \ △QBC≡△PRS,$$
$$∴ \ QQ’=PD. \qquad [1]$$
Since $AC∥RS$,
$$RR’=DP’. \qquad [2]$$
From $[1]$ and $[2]$,
$$PP’-PD=DP’,$$
$$∴ \ PP’-QQ’=RR’.$$
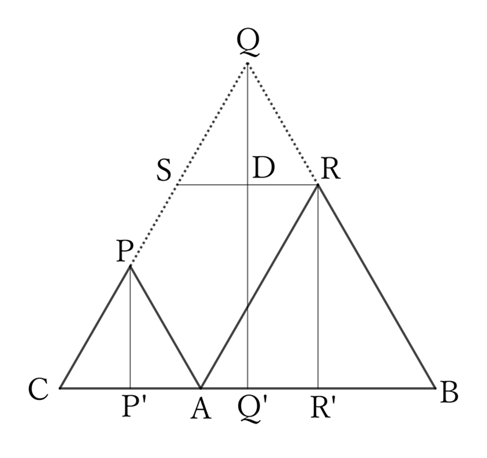
If you place $C$ on the extension of $BA$,
$$QQ’-PP’=RR’.$$
$$∴ \ |PP’-QQ’ |=RR’.$$
Hence, no matter where you place $C$ on the extension of $AB$ (or $BA$), the absolute value of the difference of the heights of $△ACP$ and $△BCQ$ is constant.
$ $
$ $
$ $
Reference Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.36.