Problem
In a quadrilateral $ABCD$, when opposite $∠A$ and $∠C$ are equal, the bisectors of another pair of opposite $∠B$ and $∠D$ are parallel to each other.
$$ $$
$$ $$
$\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$ $\downarrow$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
$$ $$
Solution
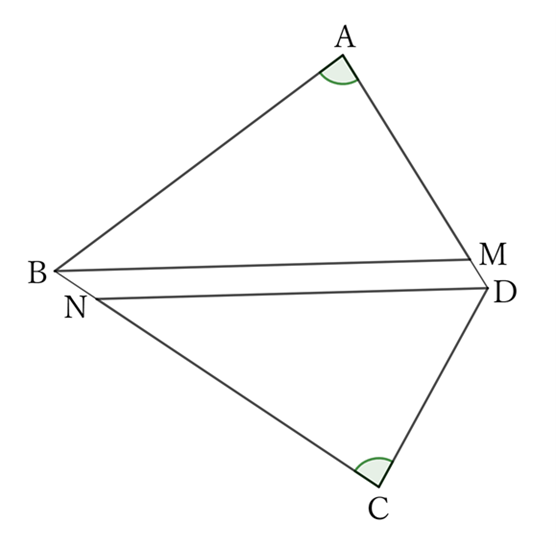
Let $BM$ and $DN$ be the bisectors of $∠B$ and $∠D$, respectively.
For a quadrilateral $ABCD$,
$$∠A+∠B+∠C+∠D=4∠R.$$
However, since $∠A=∠C$,
$$2∠A+∠B+∠D=4∠R. \qquad [1]$$
For $△ABM$,
$$∠A+∠ABM+∠AMB=2∠R,$$
$$∴ \ ∠A+\frac{1}{2}∠B+∠AMB=2∠R, \qquad \left( ∵ ∠ABM=\dfrac{1}{2}∠B \right)$$
$$∴ \ 2∠A+∠B+2∠AMB=4∠R. \qquad [2]$$
From $[1]$ and $[2]$,
$$2∠AMB=∠D,$$
$$∴ ∠AMB=\frac{1}{2} ∠ D. \qquad [3]$$
Since $DN$ is the bisector of $∠D$,
$$∠ADN=\frac{1}{2}∠D. \qquad [4]$$
From $[3]$ and $[4]$,
$$∴ \ BM∥DN.$$
$ $
$ $
$ $
Reference Teiichiro Sasabe (1976) The Encyclopedia of Geometry (2nd edition), Seikyo-Shinsha, p.42.